Question

Which value must be added to the expression x2 + x to make it a perfect-square trinomial?

A. 1/4
B. 1/2
C. 1
D. 4

Answer 1

Simple...

you have: Which value must be added to the expression
`x^(2) +x`

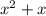
to make it a perfect-square trinomial?


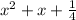
`x^(2) +x+ (1)/(4)` (Answer)

A perfect square is a whole number that is the square of an integer..-->>>

EX: 16 is a perfect square because 4*4=16


`x^(2) +x+ (1)/(2)`-->>(No)

This expression can't be factored with rational numbers, nor is the polynomial factorable with rational numbers.


`x^(2) +x+1`-->>(No)

This expression can't be factored with rational numbers, nor is the polynomial factorable with rational numbers.


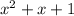
`x^(2) +x+4`-->>(No)

This expression can't be factored with rational numbers, nor is the polynomial factorable with rational numbers.

Thus, your answer.

Answer 2

we know that

Perfect square trinomials are quadratics which are the results of squaring binomials

so

`x^(2) +2ax+a^(2) =(x+a)^(2)`

we have

`x^(2) + x`

Divide the coefficient of the term x by
`2`

`=(1)/(2)`

`(x+(1)/(2) )^(2) = x^(2)+2x*(1)/(2) +(1)/(4) \\\\ (x+(1)/(2) )^(2) = x^(2)+x +(1)/(4)`

therefore

the answer is the option

A. 1/4