Answer with Step-by-step explanation:
We are given that in triangle PQR
QS bisect the angle PQR

We have to prove that
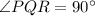

Because QS bisect the angle PQR.
Therefore,
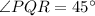
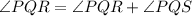
Substitute the values then we get
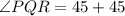
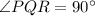
Hence, triangle PQR is right triangle because one angle of triangle is of 90 degrees.
Hence, proved.