Question

Write each fraction as an expression using a negative exponent other than negative one. The first box is for the base and the second box is for the exponent. Leave your answers in exponential form.

Answer 1

We have from the question the following fraction:

`(1)/((-5)^7)`

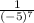
And we have to write this fraction as an expression using a negative exponent.

Then to achieve that, we can proceed as follows:

1. We know that one of the exponent rules is as follows:

`x^(-m)=(1)/(x^m)`

And it is called the Negative Exponent Rule.

2. Then we have that:

`(1)/((-5)^7)=(-5)^(-7)`

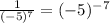
We can notice that we ended up with an expression in an exponential form whose base is (-5) and its exponent is (-7).

Therefore, in summary, the given expression is equivalent to another expression using a negative exponent. Then we can rewrite the original expression as:

`(-5)^(-7)`

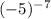
The first box is for the base: -5

The second box is for the exponent: -7