Question

Write the equation in standard form for the circle passing through (11, 2) centered at theorigin

Answer 1

Explanation

We are given that a circle passes through the coordinate (11,2) and is centered at the origin. This implies that:

`\begin{gathered} (x,y)\rightarrow(11,2) \\ (a,b)\rightarrow(0,0) \end{gathered}`

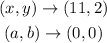
The standard form of a circle centered at the origin is given as:

`\begin{gathered} x^2+y^2=r^2 \\ where \\ r=radius \end{gathered}`

We shall calculate the radius of the circle with the above formula as:

`\begin{gathered} x^2+y^2=r^2 \\ 11^2+2^2=r^2 \\ 121+4=r^2 \\ r^2=125 \end{gathered}`

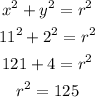
Therefore, the equation of the circle required is:

`\begin{gathered} x^2+y^2=r^2 \\ x^2+y^2=125 \end{gathered}`

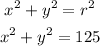
Hence, the answer is x² + y² = 125.