Question

5. Mike likes to canoe. He can paddle 150 feet per minute. He is planning a river trip that will take him to adestination about 30,000 feet upstream (that is, against the current). The speed of the current will workagainst the speed that he can paddle.a. Mike guesses that the current is flowing at a speed of 50 feet per minute. Assuming this is correct, how longwill it take for Mike to reach his destination? (1 point)b. Mike does not really know the speed of the current Fill in the table showing the time it will take him to reachhis destination for different speeds: (4 points)Speed of Current(feet per minute)Mike's Speed(feet per minute)Time for Mike to travel30,000 feet (minutes)050100140149

Answer 1

Mike speed: m

Current speed: c

Upstream speed: u

When he is traveling against the current the total speed is:

`u=m-c`

______________________

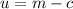
a. if the current is flowing at a speed of 50 feet per minute, the total speed of Mike upstream is:

`\begin{gathered} u=150ft/\min -50ft/\min \\ u=100ft/\min \end{gathered}`

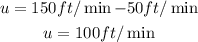
As the total distance is 30,000 ff, the time tath takes for Mike to reach his destination is:

`\begin{gathered} \text{speed}=\frac{\text{distance}}{\text{time}} \\ \\ \text{time}=\frac{\text{distance}}{\text{speed}} \\ \\ \text{time}=(30000ft)/(100ft/\min)=300\min \end{gathered}`

________________________

b. Speed of current: c

Mike's speed: u

Time for Mike to travel 30,000feet: t

____________________

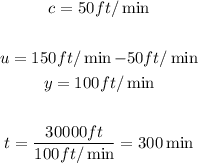
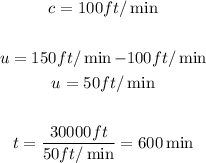
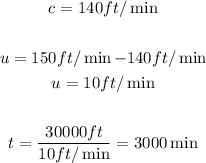
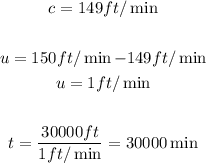
`\begin{gathered} c=0ft/\min \\ \\ u=150ft/\min -0ft/\min \\ u=150ft/\min \\ \\ t=(30000ft)/(150ft/\min)=200\min \end{gathered}`

_____________________

`\begin{gathered} c=50ft/\min \\ \\ u=150ft/\min -50ft/\min \\ y=100ft/\min \\ \\ t=(30000ft)/(100ft/\min)=300\min \end{gathered}`

___________________

`\begin{gathered} c=100ft/\min \\ \\ u=150ft/\min -100ft/\min \\ u=50ft/\min \\ \\ t=(30000ft)/(50ft/\min)=600\min \end{gathered}`

_____________________

`\begin{gathered} c=140ft/\min \\ \\ u=150ft/\min -140ft/\min \\ u=10ft/\min \\ \\ t=(30000ft)/(10ft/\min)=3000\min \end{gathered}`

_____________________

`\begin{gathered} c=149ft/\min \\ \\ u=150ft/\min -149ft/\min \\ u=1ft/\min \\ \\ t=(30000ft)/(1ft/\min)=30000\min \end{gathered}`

_____________

`\begin{gathered} c=s\text{ ft/min} \\ \\ u=150ft/\min -s\text{ ft/min} \\ \\ t=\frac{30000ft}{150ft/\min -s\text{ ft/min}} \end{gathered}`