Answer:
the magnitude of Vpg = 493.711 km/h
Step-by-step explanation:
given data
speed Vpg = 560 km/h
speed Vwg = 80 km/h
solution
we get here magnitude of the plane velocity w.r.t. ground is
we know that the Vpg = Vpw + Vwg .....................1
writing the component of the velocity that is
Vpw = (0 km/h î - 560 km/h j )
Vwg = (80 cos 45 km/h î + 80 sin 45 km/h j)
adding these
Vpg = (0+80 cos 45 km/h ) î + ( -560 + 80 sin 45 km/h j)i
Vpg = (42.025 ) î (-491.92 km/h)j
now we take magnitude
the magnitude of Vpg =
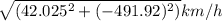
the magnitude of Vpg = 493.711 km/h