Question

Given the functions, f(x) = x3 + 2 and g(x) = x - 9, perform the indicated operation. When applicable, state the domain restriction.

Answer 1

i hope this helps you

Answer 2

Option 2 is correct.

Explanation:

We are given the functions,

`f(x)=x^3+2`

`g(x)=x-9`

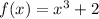
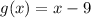
Then, we get that,

`((f)/(g))(x)=(f(x))/(g(x))`

i.e.
`((f)/(g))(x)=(x^3+2)/(x-9)`

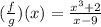
Thus, the quotient function is
`(x^3+2)/(x-9)`.

Since, the functions in the numerator and denominator are polynomial.

So, they both are defined for all real numbers.

But, as the quotient function is not defined for x= 9.

Thus, the domain for the quotient function is 'Set of real numbers excluding 9'.

Hence, option 2 is correct.