Question

Suppose that $3000 is placed in a savings account at an annual rate of 10.2%, compounded monthly. Assuming that no withdrawals are made, how long will it take for the account to grow to $3738? Do not round any Intermediate computations, and round your answer to the nearest hundredth.

Answer 1

`2.17\text{years}`

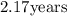
Explanations:

The formula for calculating compound amount is expressed according to the formula;

`\begin{gathered} A=P(1+(r)/(n))^(nt) \\ \end{gathered}`

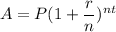
where;

P is the principal (amount saved)

A is the compounded amount

t is the time (in years)

r is the rate (in decimal)

n is the compounding time

Given the following parameters

A = $3738

P = $3000

r = 10.2% = 0.102

n = 12 (compounded monthly)

Substitute the given parameters into the formula to get the required time.

`3738=3000(1+(0.102)/(12))^(12t)`

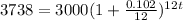
Make "t" the subject of the formula as shown;

`\begin{gathered} (3738)/(3000)=(1+0.0085)^(12t) \\ 1.246=(1.0085)^(12t) \\ \end{gathered}`

Take the natural logarithm of both sides

`\begin{gathered} \log 1.246=12t\log (1.0085) \\ 12t=(\log 1.246)/(log1.0085) \\ 12t=(0.095518)/(0.003676) \\ 12t=25.9913 \\ t=(25.9913)/(12) \\ t=2.1659 \\ t\approx2.17\text{years} \end{gathered}`

This shows that it will take 2.17 years for the account to grow to $3738