The given equation is,
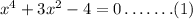
Let
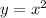
Then, equation (1) becomes,

The general form of a quadratic equation can be written as,

Comparing equations (2) and (3), we get a=1, b=3 and c=-4.
Hence, the solution for equation (2) can be calculated using discriminant method as
![\begin{gathered} y=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ y=\frac{-3\pm\sqrt[]{3^2-4*1*(-4)}}{2*1} \\ y=\frac{-3\pm\sqrt[]{9+16}}{2} \\ y=\frac{-3\pm\sqrt[]{25}}{2} \\ y=(-3\pm5)/(2) \\ y=(-3+5)/(2)\text{ or y=}(-3-5)/(2) \\ y=(2)/(2)\text{ or }y=(-8)/(2) \\ y=1\text{ or }y=-4 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/wcdjif4qqctkg59zk2ti.png)
We had defined y as,

Put y=1 and y=-4 in the above equation to find the values of x.
Putting y=1,
![\begin{gathered} x^2=1 \\ x=\pm\sqrt[]{1} \\ x=\pm1 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/121lw5v3ns5oy5b6lcd3.png)
Putting y=-4 in equation (4),
![\begin{gathered} x^2=-4 \\ x=\pm\sqrt[]{-4} \\ x=\pm\sqrt[]{-1*4} \\ x=\pm2*\sqrt[]{-1} \\ As\text{ }\sqrt[]{-1}=i, \\ x=\pm2i \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/o1025drf7yxvbpfqy4vn.png)