Question

A sailboat leaves port and sails 12 kilometers west and then 9 kilometers north. The sailboat is now kilometers from port

Answer 1

Answer: The sailboat is at a distance of 15 km from the port.

Step-by-step explanation: Given that a sail boat leaves port and sails 12 kilometers west and then 9 kilometers north.

We are to find the distance between the sailboat from the port in kilometers.

Since the directions west and north are at right-angles, we can visualize the movement of the sailboat in the form of a right-angled triangle as shown in the attached figure.

The sailboat moves leaves the port at P and reach O after sailing 12 km west. From point O, again it moves towards north 9 km and reach the point S.

PS = ?

Using the Pythagoras theorem, we have from right-angled triangle SOP,

`PS^2=OS^2+OP^2=9^2+12^2=81+144=225\\\\\Rightarrow PS=15~\textup{km}.`

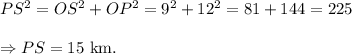
Thus, the sailboat is at a distance of 15 km from the port.