Question

1. Find the specified quantities in the following figures. You may use the following formulas: thecircumference of a circle is given by C = 2nr(C) FIND THE AREA OF FIGURE C

Answer 1

#C

From the given figure we can see a rectangle with a semicircle cut from it

Then to find the area of the figure we will subtract the area of the semicircle from the area of the rectangle

Since the length of the rectangle is 6m

Since the width of the rectangle is 4 m

Since the rule of the area of the rectangle is

`A=L* W`

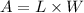
Then substitute L by 6 and W by 4

`\begin{gathered} A_R=6*4 \\ A_R=24\text{ m}^2 \end{gathered}`

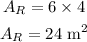
Since the radius of the semicircle is 2 m

Since the rule of the area of the semicircle is

`A=(1)/(2)\pi r^2`

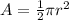
Then substitute r by 2

`\begin{gathered} A_(semicircle)=(1)/(2)(\pi)(2^2) \\ A_(semicircle)=(1)/(2)(\pi)(4) \\ A_(semicircle)=2\pi \end{gathered}`

Now, we will subtract the area of the semicircle from the area of the rectangle to find the area of the figure

`\begin{gathered} A_F=A_R-A_(semicircle) \\ A_F=24-2\pi \\ A_F=(24-2\pi)\text{ m}^2 \end{gathered}`

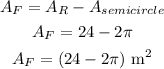
Then the exact area of the figure is (24 - 2pi) m^2

If the value of pi = 3.14, then

The area of the figure is

`\begin{gathered} A_F=24-2(3.14) \\ A_F=17.72\text{ m}^2 \end{gathered}`

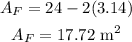
The area of the figure is 17.72 m^2