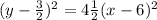Question

Derive the equation of the parabola with a focus at (6,2) and a directrix of y=1

Answer 1

distance from focus to vertex=distance from vertex to directix

from (6,2) to y=1, the distance is 1
the focus is 1/2 higher than the vertex
vertex=(6,1.5)

for
(x-h)^2=4p(y-k)
vertex=(h,k)
p=distance from vertex to directix

vertex=(6,1.5)
ditance=0.5

(x-6)^2=4(0.5)(y-1.5)
(x-6)^2=2(y-1.5) is the equation
or
y=0.5x^2-6x+19.5 or
y=0.5(x-6)^2+1.5

Answer 2

`(y-(3)/(2))^2=2(x-6)^2` equation of parabola

Explanation:

Focus is (h,k+p)=(6,2)

And directrix y=k-p=1

When comparing the values we get

h=6 and

k+p=2 (a)

k-p=1 (b)

For solving (a) and (b) we substitute k=2-p in (b) we get:

`2-p-p=1`

`2-2p=1`

`p=(1)/(2)`

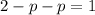
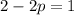
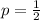
Hence, put
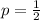`p=(1)/(2)` in k=2-p we get:

`k=2-(1)/(2)`

`k=(3)/(2)`

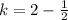
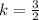
Now, we have general equation as:

`(y-k)^2=4p(x-h)^2`

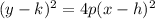
On substituting the values in general equation we get:

`(y-(3)/(2))^2=4(1)/(2)(x-6)^2`

`(y-(3)/(2))^2=2(x-6)^2`