Question

For what value of x and y must this quadrilateral be a parallelogram? Find the lengths of the sides

Answer 1

- In order for the quadrilateral to be a parallelogram, the opposite sides of the quadrilateral must be parallel and equal in length.

- Thus, we can say:

`\begin{gathered} BC=AD \\ AB=CD \end{gathered}`

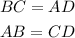
- This means we can equate the expression of BC to that of AD and the expression of AB to that of CD.

- Let us perform this operation below:

`\begin{gathered} BC=AD \\ 5x+4y=7x-2 \\ \text{Let us simplify this expression as follows:} \\ \\ \text{Subtract 7x from both sides} \\ 5x-7x+4y=-2 \\ -2x+4y=-2\text{ (Equation 1)} \\ \\ AB=CD \\ 2y+x=3y+5 \\ \text{Subtract both 3y from both sides } \\ 2y-3y+x=5 \\ -y+x=5\text{ (Equation 2)} \end{gathered}`

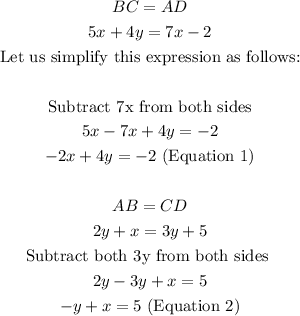
- Thus, let us solve these two equations simultaneously.

- We shall apply the substitution method.

`\begin{gathered} -2x+4y=-2\text{ (Equation 1)} \\ -y+x=5\text{ (Equation 2)} \\ \\ \text{From Equation 2,} \\ x=5+y \\ \\ \text{Insert this expression for }x\text{ into Equation 1} \\ -2(5+y)+4y=-2 \\ \text{Expand the bracket and simplify} \\ -10-2y+4y=-2 \\ -10+2y=-2 \\ \text{Add 10 to both sides} \\ 2y=-2+10=8 \\ \\ \text{Divide both sides by 2} \\ (2y)/(2)=(8)/(2) \\ \\ \therefore y=4 \\ \\ \text{Next, we can solve for x by substituting the value of y into any of the Equations} \\ \text{Substituting }y=4\text{ into Equation 2} \\ -y+x=5 \\ \text{put }y=4 \\ -4+x=5 \\ \text{Add 4 to both sides} \\ x=5+4 \\ \\ \therefore x=9 \end{gathered}`

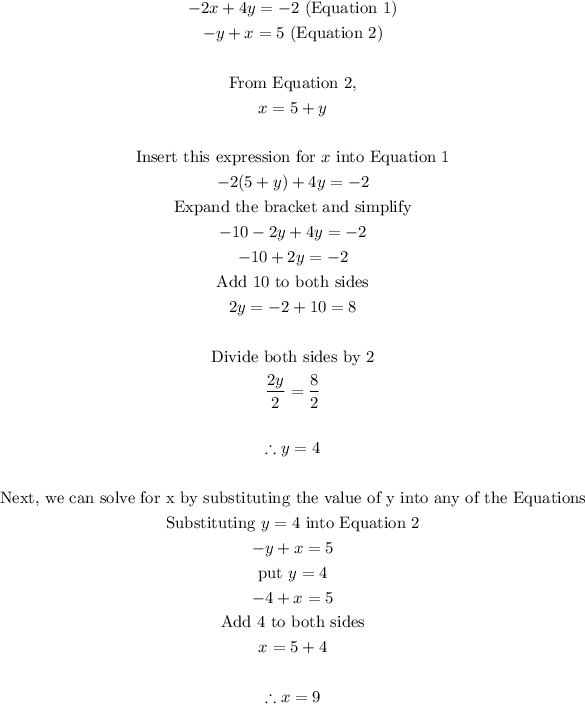
Final Answer

The value of x is 9

The value of y is 4

-