Question

What is the domain of the function

y=3√6x+42 A. x ≥ 0
B. x ≤ 7
C. x ≥ –6
D. x ≥ –7

Answer 1

The domain of the function:
y = 3 √ ( 6 x + 42 )
6 x + 42 ≥ 0
6 x ≥ - 42
x ≥ - 42 : 6
x ≥ - 7
Answer:
The domain is D ) x ≥ - 7

Answer 2

Answer: The correct option is (D)
`x\geq -7.`

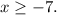
Step-by-step explanation: We are given to find the domain of the following function:

`y=3√(6x+42)~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

The domain of a function y = f(x) is the set of all possible x-values which will make the function defined or will output real y-values.

That is, for the function (i), the domain will be given by all those values of x for which
`3√(6x+42)` is real.

Now, the values of y will be real only if the expression under square root is greater than or equal to 0.

Therefore, the domain of function (i) is

`√(6x+42)\geq0\\\\\Rightarrow 6x+42\geq 0\\\\\Rightarrow 6x\geq -42\\\\\Rightarrow x\geq -(42)/(6)\\\\\Rightarrow x\geq -7.`

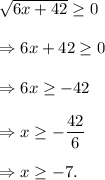
Thus, the domain of the given function is
`x\geq -7.`

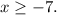
Option (D) is CORRECT.