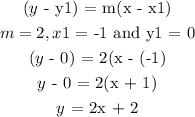Question

Referring to the figure, write the equation in standard form of the horizontal line through the point (-1,0)

Answer 1

The equation of the line that passes through (-1, 0) is y = 2x + 2

Explanation

Given information

The given points on the graph are (-1, 0) and (0, 2)

From the given points, we can deduce the below data

x1 = -1

y1 = 0

x2 = 0

y2 = 2

The next step is to find the slope between the two points

`\text{slope = }\frac{\text{ rise}}{\text{ run}}`

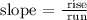
Where

rise = y2 - y1

run = x2 - x1

`\begin{gathered} \text{slope = }\frac{y2\text{ - y1}}{x2\text{ - x1}} \\ \text{slope = }\frac{\text{ 2 - 0}}{0\text{ - (-1)}} \\ \text{slope = }\frac{2}{0\text{ + 1}} \\ \text{slope = }(2)/(1) \\ \text{slope = 2} \end{gathered}`

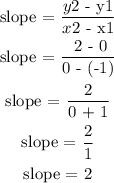
From the above equation, you will see that the slope between the two lines is 2

The next process is to find the equation of the line that passes through (-1, 0)

Recall that,

`y\text{ = mx + b}`

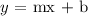
where,

m is the slope of the line

b is the intercept of the y-axis

For a given point, we will be using the formula below

`\begin{gathered} (y\text{ - y1) = m(x - x1)} \\ m=2,x1\text{ = -1 and y1 = 0} \\ (y\text{ - 0) = 2(x - (-1)} \\ y\text{ - 0 = 2(x + 1)} \\ y\text{ = 2x + 2} \end{gathered}`