Question

What is the cube root of 27a^12

Answer 1

Cube root of 27 a^12 will be: 3 a^4.

Answer 2

Cube-root defines: A number is a special value that, when used in a multiplication three times, gives that number.

Its symbol is denoted by:
`\sqrt[3]{}`

USing Law of radical:

1.
`\sqrt[n]{ab}= \sqrt[n]{a} \cdot \sqrt[n]{b}`

2.
`\sqrt[n]{x^n} =(\sqrt[n]{x} )^n =\sqrt[n]{x^n} =x`

To find the cube root of
`27a^(12)` ;

By the definition of cube root

`\sqrt[3]{27 a^(12)}` =
`\sqrt[3]{27} \cdot \sqrt[3]{a^(12)}` [Using [1]]

we can write 27 as
`3 * 3 * 3 = 3^3` and
`a^(12) = (a^4)^3`

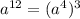
then;

`\sqrt[3]{27 a^(12)}` =
`\sqrt[3]{3^3} \cdot \sqrt[3]{a^(4)^3}`

=
`3 \cdot a^4` [ Using [2] ]

therefore, the cube root of
`27a^(12)` is
`3a^4`