Answer:
25%
Explanation:
Given that prices of a gallon of milk at various stores in one town have a mean of $3.96 with a standard deviation of $0.12.
When sales lie between 3.72 and 4.20 dollars we get
we have 3.72 = 3.96-2 (0.12) and
4.20 = 3.96+2(0.12)
Hence simply we can write
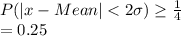
i.e. 25% is the minimum percentage of stores that sell a gallon of milk for between $3.72 and $4.20