Question

Write an inequality that represents the following problem. There are two cell phone plans available from Cell Phones R Us company. Plan A charges $30 a month plus $0.01 for every text message. Plan B charges $20 a month plus $0.05 for every text message. How many text messages do you need to send in order for Plan A to be cheaper than Plan B?

Answer 1

Minimum number of messages to be sent is 251 for Plan A to be cheaper than Plan B.

Explanation:

Let the text messages needed to be sent in order for Plan A to be cheaper than Plan B be N

Therefore total cost of sending text messages in case of Plan A is

`C_A=\$ (30+0.01N)`

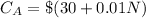
and total cost of sending text messages in case of Plan B is

`C_B=\$ (20+0.05N)`

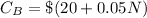
Now for Plan A to be cheaper than Plan B ,
`C_A < C_B`

`\therefore (30+0.01N)< (20+0.05N)=>N> 250`

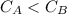
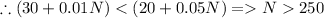
Thus for Plan A to be cheaper than Plan B more than 250 messages needed to be sent . Minimum number of messages to be sent is 251 for Plan A to be cheaper than Plan B.

Answer 2

if PLAN A is cheaper than PLAN B then,
P (A) < P (B)
30+0.01t < 20+0.05t (subtract 0.05t from both sides)
29.95-0.04t < 19.95 (subtract 29.95 from both sides)
-0.04t < -10 (divide both sides by -0.04)
t > 250
So plan A is cheaper than plan B when you send more than 250 texts.
Hope it helps.