Answer:
a) Range = 1.39
b) the sample variance is 0.1597
c) the sample standard deviation is 0.3996
Explanation:
Given the data in the question;
a) Find the range.
To determine the range, we simple subtract the smallest value from the largest value. i.e
Range = largest value - smallest value
from data set; our smallest is 0.53 while our largest value is 1.92
so
Range = 1.92 - 0.53 = 1.39
b) Find the sample variance.
To determine our variance, we use the following formula;
∑(
 -
-
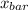 )² / n - 1 = [ ∑
)² / n - 1 = [ ∑
 ²/n-1 ] - [
²/n-1 ] - [
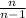 (
(
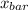 )²]
)²]
where
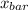 = ∑
= ∑
 /n
/n
n is sample size = 14 so lets calculate ∑

∑
 = 0.99 + 1.92 + 1.23 + 0.85 + 0.65 + 0.53 + 1.41 + 1.12 + 0.63 + 0.67 + 0.69 + 0.60 + 0.60 + 0.66
= 0.99 + 1.92 + 1.23 + 0.85 + 0.65 + 0.53 + 1.41 + 1.12 + 0.63 + 0.67 + 0.69 + 0.60 + 0.60 + 0.66
∑
 = 12.55
= 12.55
∑
 ² = 0.99² + 1.92² + 1.23² + 0.85² + 0.65² + 0.53² + 1.41² + 1.12² + 0.63² + 0.67² + 0.69² + 0.60² + 0.60² + 0.66²
² = 0.99² + 1.92² + 1.23² + 0.85² + 0.65² + 0.53² + 1.41² + 1.12² + 0.63² + 0.67² + 0.69² + 0.60² + 0.60² + 0.66²
∑
 ² = 13.3253
² = 13.3253
so
our
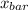 = ∑
= ∑
 /n = 12.55 / 14 = 0.8964
/n = 12.55 / 14 = 0.8964
so our Variance will be;
= [ ∑
 ²/n-1 ] - [
²/n-1 ] - [
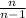 (
(
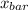 )²]
)²]
= [ 13.3253 / 14-1 ] - [
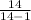 (0.8964)²]
(0.8964)²]
= 1.025 - 0.8653
= 0.1597
Therefore, the sample variance is 0.1597
c) Find the sample standard deviation.
we know that standard deviation is the square root of variance;
standard deviation = √Variance
standard deviation = √0.1597
standard deviation = 0.3996
Therefore, the sample standard deviation is 0.3996