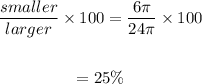Question

find the surface areas of the larger cone and the smaller cone in terms of pi. compare the surface areas using a percent. (the middle one)

Answer 1

Surface area of larger cone : 24π units^2

Surface area of smaller cone : 6π units^2

The surface area of the smaller cone is 25% of that of the larger cone.

EXPLANATION :

From the given problem,

AB = 3 is the radius of the larger cone and the slanted height is BC = 5

DE = 1.5 is the radius of the smaller cone and the slanted height is EC = 2.5

Recall the surface area of the cone :

`A=\pi r^2+\pi rL`

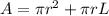
where r = radius and L = slanted height.

For the larger cone, r = 3 and L = 5

`\begin{gathered} A=\pi(3)^2+\pi(3)(5) \\ A=24\pi \end{gathered}`

For the smaller cone, r = 1.5 and L = 2.5

`\begin{gathered} A=\pi(1.5)^2+\pi(1.5)(2.5) \\ A=6\pi \end{gathered}`

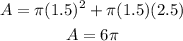
Comparing the surface areas :

The area of the smaller cone compared to the larger cone is :

`\begin{gathered} (smaller)/(larger)*100=(6\pi)/(24\pi)*100 \\ \\ =25\% \end{gathered}`