Question

express each sum using summation notation, use 1 as the lower limit of summation and i for the index of summation

Answer 1

Answer:
`\sum_{i\mathop{=}1}^(14)\frac{i}{i+\text{ 1}}`

Step-by-step explanation:

Given:

`(1)/(2)+\text{ }(2)/(3)+(3)/(4)+\text{ . . . +}\frac{14}{14+\text{ 1}}`

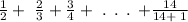
To find:

To express the sum using summation notation using 1 as the lower limit and i for the index notation

Summation notation is given as:

`\begin{gathered} \sum_{i\mathop{=}lower\text{ limit}}^{upper\text{ limit}}function \\ \\ We\text{ need to find the function} \\ from\text{ the expanded number, we see the numerator for first number = 1} \\ second\text{ number's numerator = 2} \\ 3rd\text{ number's numerator = 3} \\ last\text{ number's numerator = 14} \\ \\ This\text{ means our upper bound = 14 and lower bound = 1} \end{gathered}`
`\begin{gathered} \sum_{i\mathop{=}1}^(14)function \\ The\text{ denominator of first number = 2 = 1 + 1} \\ Since\text{ i = 1, it will be i + 1} \\ The\text{ denominator of the 2nd number = 3 = 2 + 1} \\ since\text{ i = 2, it will be i + 1} \\ The\text{ denominator of the 3rd number = 4 = 3 + 1} \\ since\text{ i = 3, it will be i + 1} \\ The\text{ denominator of the 14th number = 15 = 14 + 1} \\ since\text{ i = 14, it will be i + 1} \end{gathered}`

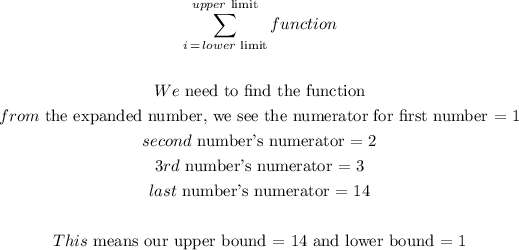
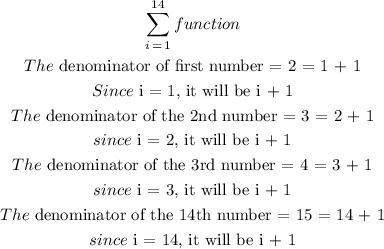
This means the rule of the denominator = i + 1

The rule of the numerator = i

`The\text{ function becomes: }\frac{i}{i\text{ + 1}}`

The summation notation for the sum will be:

`\sum_{i\mathop{=}1}^(14)\frac{i}{i+\text{ 1}}`