Question

A ratio of two complementary angles is 3:7 find the measures of both angles

Answer 1

Given the word problem, we can deduce the following information:

1. The ratio of the two complementary angles is 3:7.

To determine the measures of both angles, we must note first that complementary angles are two angles whose measures add up to 90°. So our equation would be:

`3x+7x=90`

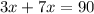
We simplify the equation above:

`\begin{gathered} 3x+7x=90 \\ 10x=90 \\ x=(90)/(10) \\ x=9 \end{gathered}`

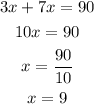
We plug in x=9 into 3x to get the first angle:

Angle 1 = 3x = 3(9) = 27

Then, we plug in x=9 into 7x to get Angle 2:

Angle 2= 7x=7(9)=63

Therefore, the measures of both angles are 27° and 63°.