Given:
The given geometric sequence is 3, 12, 48, 192, ...
Required:
To find the 9th term in the given geometric sequence.
Step-by-step explanation:
The first term is
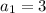
Common ratio is
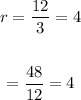
The formula for nth term in geometric sequence is
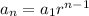
Here
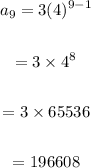
Final Answer:
The 9th term is 196608.