To solve the exercise, we can consider the following system of two linear equations with two unknown variables:
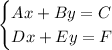
If the pair (A,B) is not proportional to the pair (D,E), then there is only one solution. That is, there is no such number k that
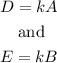
In other words, we have to verify the following:
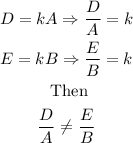
In this case, we have:
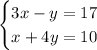

Therefore, since the pair (3, -1) is not proportional to the pair (1,4), then there is only one solution for the given system of linear equations.