Solution:
Concept:
The volume of the triangular pyramid will be calculated using the formula below

In this case, the base is a triangle...Therefore, the area of the base will be calculated using the formula below

Step 1:
Calculate the area of the base using the formula above

By substituting the values, we will have
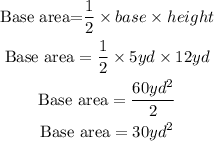
Step 2:
Calculate the volume of the triangular based pyramid using the formula below

By substituting the values, we will have
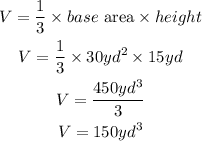
Hence,
The volume of the triangular based pyramid is = 150 yd³