Question

Reduce the fraction. Hint: Factor the numerator and the denominator first.


`a^2+2ac+c^2/a^2+ac-ax-cx`

Answer 1

`((a+c))/((a-x))`

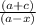
Explanation:

Ok, first let's factor out the numerator:

Factor: Numerator

We can use the special binomial product (a + b)² = a² + 2ab + b²

• a² + 2ac + c²
• (a + c)²

Factor: Denominator

We can factor by grouping

• a² - ax + ac - cx
• a(a - x) + c(a - x)
• (a - x)(a + c)

Simplify:

We can remove like terms.

• 
  `((a+c)(a+c))/((a-x)(a+c))` <= Cancel out (a+c)
• 
  `((a+c))/((a-x))`

-Chetan K

Answer 2

• (a + c) / (c - x)

Explanation:

Simplify:

• (a² + 2ac + c²) / (c² + ac - ax - cx) =
• (a + c)² / (c(a + c) - x(a + c)) =
• (a + c)² / (a + c)(c - x) = Cancel out (a + c)
• (a + c) / (c - x)