Question

01:55:23ABased on the diagram, which expresses all possiblelengths of segment AB?OAB = 2527EN27 81Save and ExitSubmit

Answer 1

To get the possible length of segment AB from the triangle given, then we use the triangle inequality theorem.

The Triangle inequality theorem states that the sum of any 2 sides of a triangle must be greater than the measure of the third side.

From this theorem, we sum the two sides given

Given:

`\begin{gathered} AC=27 \\ BC=54 \end{gathered}`
`\begin{gathered} AC+BC=27+54 \\ AC+BC=81 \end{gathered}`

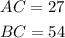
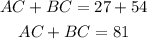
Applying the triangle inequality theorem, the sum of any 2 sides of a triangle must be greater than the measure of the third side.

This means AC + BC > AB

81 > AB. This also means AB < 81

From the options given, AB = 25 is not a possible value because adding it to side AC gives 52. Hence, when AB = 25, AB + AC < BC (52 < 54) and does not obey the triangle inequality theorem.

Also, AB < 27 or AB > 81 will not be a possible value.

Therefore, the expression for all possible lengths of segment AB of the triangle is

27 < AB < 81 as it will satisfy the triangle inequality theorem.