We are given that a number is added to the numerator of 5/6. If "x" is the number then this can be written mathematically as:
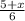
we also told that twice this number is added to the denominator, this can be written mathematically as:
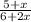
We are also told that the result of this operation of 3/5, therefore, we have:

We get an equation with one variable. To solve this equation we will cross multiply the equation, like this:
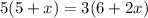
Now we will apply the distributive property on both parentheses:
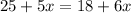
Now we subtract 6x from both sides:
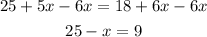
Now we subtract 25 from both sides:
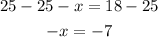
Now we multiply both sides by -1:
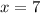
Therefore, the number is 16.
Let's replace the value of "x" in the expression: