Question

13.-x - y - 2z = 9-2x +2y -2z = -8x - y + z = 5

Answer 1

The System of equation is the following:

`\begin{gathered} -x-y-2z=9 \\ -2x+2y-2z=-8 \\ x-y+z=5 \end{gathered}`

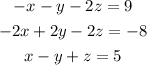
First, notice that we can simplify the second equation by dividing it by 2:

`\begin{gathered} (-2x+2y-2z=-8)\, /\, 2 \\ -(2x)/(2)+(2y)/(2)-(2z)/(2)=-(8)/(2) \\ -x+y-z=-4 \end{gathered}`

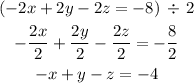
Now, we have:

`\begin{gathered} -x-y-2z=9 \\ -x+y-z=-4 \\ x-y+z=5 \end{gathered}`

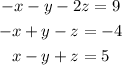
Now, notice that if we change the sign of the whole second equation:

`\begin{gathered} -(-x+y-z=-4) \\ x-y+z=4 \end{gathered}`

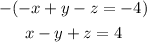
We have the second and the third equations almost the same:

`\begin{gathered} x-y+z=4 \\ x-y+z=5 \end{gathered}`

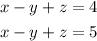
However, since the left side of them are exactly the same, the right side would have to be the same, by they aren't, because one is equal to "4" and the other is equal to "5".

So, because of this, there is no solution for this system of equations.