Question

Answer is given, explain the full process including final steps

Answer 1

Given the curve:

`y=(18)/(x^2+2)`

You need to find the equation for the tangent to that curve at the point:

`(1,6)`

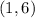
Then, you need to follow these steps:

1. Derivate the function given in the exercise using these Derivative Rules:

`(d)/(dx)((1)/(u(x)))=-(u^(\prime)(x))/(u(x)^2)`
`(d)/(dx)(x^n)=nx^(n-1)`
`(d)/(dx)(k)=0`

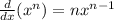
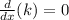
Where "k" is a constant.

Then, you get:

`=18\cdot((x^2+2)^(\prime))/((x^2+2)^2)`
`=-18\cdot((x^2+2)^(\prime))/((x^2+2)^2)`
`=-18\cdot(2x)/((x^2+2)^2)`
`y^(\prime)=-(36x)/((x^2+2)^2)`

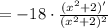
2. Substitute this value of the x-coordinate of the given point into the derivated function and then evaluate, in order to find the slope of the line:

`m=-(36(1))/((1^2+2)^2)=-(36)/(9)=-4`

4. The Point-Slope Form of the equation of a line is:

`y-y_1=m(x-x_1)`

Where "m" is the slope of the line and this point is on the line:

`(x_1,y_1)`

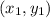
In this case:

`\begin{gathered} m=-4 \\ x_1=1 \\ y_1=6 \end{gathered}`

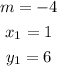
Therefore, you can substitute values:

`y-6=-4(x-1)`

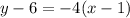
5. Convert the equation from Point-Slope Form to Slope-Intercept Form.

The equation of a line in Slope-Intercept Form is:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept.

Then, by solving for "y", you get:

`y-6=(-4)(x)-(-4)(1)`

`y-6=-4x+4`
`y=-4x+4+6`
`y=-4x+10`

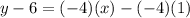
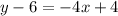
Hence, the answer is:

1. Derivate the function.

2. Find the slope of the tangent line by substituting the x-coordinate of the given point into the function derivated.

3. Write the equation of the tangent line in Point-Slope Form using the given point and the slope.

4. Rewrite the equation in Slope-Intercept Form by solving for "y".

Equation for the tangent of the curve:

`y=-4x+10`