Question

A family’s well pumps water into a tank at a rate of 10 gal/min. In the morning, the tank has 1700 gal. Which equation models the amount of water y in the tank after the well pumps for x min?

a. y=-10x-1700
b. Y= -10x + 1700
c. Y= 10x + 1700
d. Y= 10x - 1700

Answer 1

I would say the answer is D. Y=10x -1700. I think this because if you do the work: which changes the value of -1700 to 1700 when switching to other side leaving the equation 1700=10x so you just divide to find your final answer which is 170 minutes to get 1700 gallons in total. I hope this helps!

Answer 2

Option C is correct

`y = 10x+1700`

Explanation:

Using Slope-intercept form:

The equation of line is given by:

`y=mx+b` .....[1]

where,

m is the slope or rate of the line.

b is the y-intercept.

As per the statement:

Here, y represents the amount of water in the tank and x be the time in min.

A family’s well pumps water into a tank at a rate of 10 gal/min.

⇒
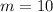`m = 10` gal/min.

It is also given that: In the morning, the tank has 1700 gal.

Initial value y(0) = b = 1700 gal

Substitute the given values in [1] we have;

`y = 10x+1700`

Therefore, an equation models the amount of water y in the tank after the well pumps for x min is,
`y = 10x+1700`