Answer:
The correct option is A.
Explanation:
It is given that a quartic function has only two real zeros.
The degree of quartic function is 4. The function has only two real roots, therefore the roots has their multiplicity.
Put -1 and -4 for x. If the value of f(x)=0 at x=-4 and x=-1 then the function have two zeros -4 and -1.

Put x=-1
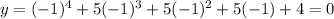
Put x=-4

Therefore option A is correct.

The value of y is 2 at x=-1 and 512 at x=-4, therefore option B is incorrect.
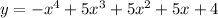
The value of y is -2 at x=-1 and -512 at x=-4, therefore option C is incorrect.
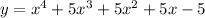
The value of y is -9 at x=-1 and -9 at x=-4, therefore option D is incorrect.