Question

If a polynomial has four terms, 3x3 + 5x + 6x2 + 10, which factoring method can be considered?

Answer 1

We can factorize the polynomial using method of grouping terms.

Explanation:

Consider the given polynomial
`3x^3 + 5x + 6x^2 + 10`

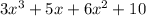
We have to find the factoring method that can be considered to solve the given polynomial.

Consider the given polynomial
`3x^3 + 5x + 6x^2 + 10`

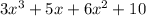
Since, the polynomial involves 4 terms,

So we can factorize the polynomial using grouping terms,

First rearranging in standard form in decreasing order of degrees of x.

`3x^3  + 6x^2 + 5x + 10`

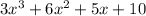
Grouping two terms together, we get,

`=\left(3x^3+6x^2\right)+\left(5x+10\right)`

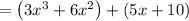
Now taking
`3x^2` common from first two and 5 from last two , we have,

`=3x^2\left(x+2\right)+5\left(x+2\right)`

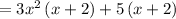
Now, taking (x+2) common, we have,

`=\left(x+2\right)\left(3x^2+5\right)`

`3x^3 + 5x + 6x^2 + 10=\left(x+2\right)\left(3x^2+5\right)`

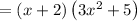
Thus, We can factorize the polynomial using method of grouping terms.