Question

A contractor wishes to build 9 houses, each different in design. In how many ways can he place these houses on a street if 6 lots are on one side of the street and 3 lots are on the opposite side?

Answer 1

Hello,

Let's put the 6 houses on side: 6P9=9*8*7*6*5*4/6! = 84

For each choice there are 3! choices pour the other side

84*3!=504

Answer 2

Answer: 362880

Explanation:

Given : A contractor wishes to build 9 houses, each different in design.

he place these houses on a street if 6 lots are on one side of the street and 3 lots are on the opposite side.

Choices to arrange first lot = 9

After first house is designed , Choices to arrange second lot = 8

After second house is designed , Choices to arrange third lot = 7

and so on.....

So the total number of ways to arrange 9 lots will be :-

`9*8*7*6*5*4*3*2*1\\\\=362880`

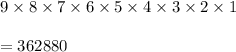
Hence, the required number of ways =362880