Question

Find the standard form of the equation of the parabola with a focus at (0, 2) and a directrix at y = -2.

Answer 1

The standard form of the equation of the parabola with a focus at (0, 2) and a directrix at y = -2 is
`y=(1)/(8)x^2`.

Explanation:

The standard form of the parabola is

`y=ax^2+bx+c`

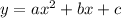
The general form of the parabola is

`(x-h)^2=4p(y-k)` ..... (1)

Where, (h,k) is vertex, (h,k+p) is focus and y=k-p is directrix.

It is given that the parabola with a focus at (0, 2) and a directrix at y = -2. It means

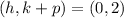
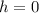
`(h,k+p)=(0,2)`

`h=0`

`k+p=2` .... (2)

`y=k-p\Rightarrow k-p=-2` .... (3)

On solving (2) and (3), we get

`k=0`

`p=2`

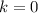
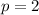
Substitute h=0, k=0 and p=2 in equation (1).

`(x-0)^2=4(2)(y-0)`

`x^2=8y`

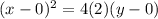
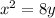
Divide both sides by 8.

`(1)/(8)x^2=y`

Therefore the standard form of the equation of the parabola with a focus at (0, 2) and a directrix at y = -2 is
`y=(1)/(8)x^2`.

Answer 2

Since the focus is at (0, 2) and directrix is y = -2

point where both of these have the same x-value will be at (0, 2) for the focus and (0, -2) for the directrix.

The vertex will also have the same x-value so it will be (0, y).

y-value is half-way between the y-value of the focus, and the y-value of the directrix at x = 0.

Directrix y-value is -2 at x = 0 and for the focus it's 2 at x = 0.

Halfway between y = -2 and y = 2 is y = 0.

So the vertex of the parabola occurs at (0, 0).

So that's x^2 = 4ay = 4(2)y = 8y.

y = 1/8*x^2

hope it helps