SOLUTION
The parabola psses through the three points
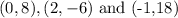
Using the form

Substitute each of the point in for x and y into the form given above
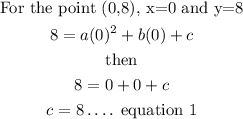
then
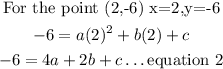
Similarly, for the last point, we have

Substitute the equation1 into equation 2 and equation 3
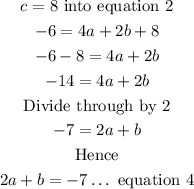
Similarly for equation 3, we have
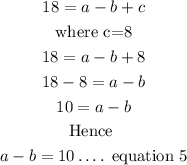
Hnece, solve equation 4 and 5 simultaneously for find the value of a and b
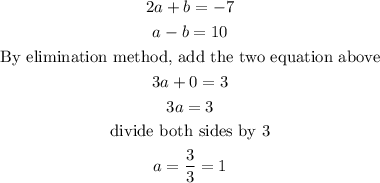
The substitute a=1 into equation 5 to obtain b
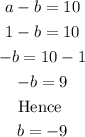
Hnece
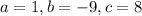
Substitute the values in the form below
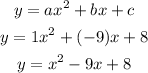
Then
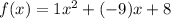
Therefore, the equation of the parabola is f(x)=1x²-9x+8
Answer: f(x)=1x²-9x+8