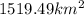Answer:
The area after 6 years is
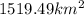
Explanation:
Given
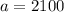 --- Initial Value
--- Initial Value
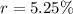 --- Rate
--- Rate
Required
Determine the area after 6 years
Because the area reduces,, the following formula will be used to solve the question.
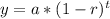
Here
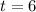
So, we have:

Convert percentage to decimal
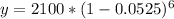
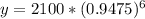

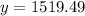 -- approximated
-- approximated
The area after 6 years is