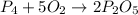Question

When 0.422g of phosphorus is burned, 0.967g of a white oxide is obtained.

a. Determine the empirical formula of the oxide
b. write a balanced equation for the reaction of phosphorus and molecular oxygen on the basis of this empirical formula

Answer 1

moles = mass / molar mass

moles P = 0.422 g / 30.97 g/mol = 0.01363 mol
moles O = (0.967 g - 0.422g) / 16.00 g/mol = 0.03406 moles

So ratio moles P : moles O
= 0.01363 mol : 0.03406 mol

Divide each number in the ratio by the smallest number

(0.01363 / 0.01363) : (0.03406 / 0.01363)
= 1 : 2.5

The empirical formula needs to be the smallest whole number ratio of atoms in the molecules. Since you have a non-whole number, multiply the ratio by the smallest number needed to make both number whole numbers. In this case x 2

2 x (1 : 2.5)
= 2 : 5

Answer 2

(a) The empirical formula of a compound is,
`P_2O_5`

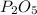
(b) The balanced chemical equation will be:

`P_4+5O_2\rightarrow 2P_2O_5`

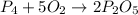
Solution : Given,

Mass of phosphorus = 0.422 g

Mass of white oxide = 0.967 g

Molar mass of phosphorus = 31 g/mole

Molar mass of oxygen = 16 g/mole

First we have to calculate the mass of oxygen.

Mass of oxygen = Mass of white oxide - Mass of phosphorus

Mass of oxygen = 0.967 - 0.422

Mass of oxygen = 0.545 g

Now convert given masses into moles.

Moles of P =
`\frac{\text{ given mass of P}}{\text{ molar mass of P}}= (0.422g)/(31g/mole)=0.0136moles`

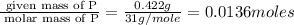
Moles of O =
`\frac{\text{ given mass of O}}{\text{ molar mass of O}}= (0.545g)/(16g/mole)=0.0341moles`

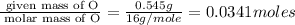
For the mole ratio, divide each value of moles by the smallest number of moles calculated.

For P =
`(0.0136)/(0.0136)=1`

For O =
`(0.0341)/(0.0136)=2.5`

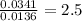
The ratio of P : O = 1 : 2.5

To make this ratio in a whole number we multiple ratio by 2, we get:

The ratio of P : O = 2 : 5

The mole ratio of the element is represented by subscripts in empirical formula.

The Empirical formula =
`P_2O_5`

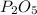
The balanced chemical equation will be:

`P_4+5O_2\rightarrow 2P_2O_5`