Question

A 10% solution of fertilizer is to be mixed with a 60% solution of fertilizer in order to get 150 gallons of a 50% solution. How many gallons of the 10% solution and 60% solution should be mixed?

Answer 1

Let the amount of gallons of 10% solution be "x", and

Let the amount of gallons of 60% solution be "y".

We need 150 gallons, thus we can write:

`x+y=150_{}`

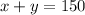
Both 10% and 60% solution needs to be mixed to produce 150 gallons of 50% solution. We can write:

`\begin{gathered} 0.1x+0.6y=0.5*150 \\ 0.1x+0.6y=75 \end{gathered}`

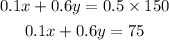
Solving for "x" in the first equation >>>

`\begin{gathered} x+y=150 \\ x=150-y \end{gathered}`

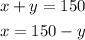
We will substitute it into the second equation and solve for "y" >>>

`\begin{gathered} 0.1x+0.6y=75 \\ 0.1(150-y)+0.6y=75 \\ 15-0.1y+0.6y=75 \\ 0.5y=60 \\ y=120 \end{gathered}`

Now, we can solve for "x" using the first equation >>>>

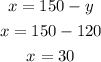
`\begin{gathered} x=150-y \\ x=150-120 \\ x=30 \end{gathered}`Answer

We need 30 gallons of 10% solution and 120 gallons of 60% solution