Question

Graph the line that passes through (-6, -4) and has a slope of 2/3. plot at least two additional points that lie on the line

Answer 1

To graph the line that passes through the point (-6,-4) and has a slope equal to 2/3, the first step is to determine its equation.

To determine the equation of the line, use the point-slope form:

`y-y_1=m(x-x_1)`

Where

(x₁,y₁) are the coordinates of one point of the line

m is the slope of the line

Replace the formula with the coordinates of the point x₁=-6 and y₁=-4, and the slope m=2/3

`\begin{gathered} y-(-4)=(2)/(3)(x-(-6)) \\ y+4=(2)/(3)(x+6) \end{gathered}`

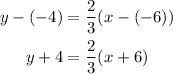
To be able to calculate two points of the line, let's write it in the slope-intercept form first:

-Distribute the multiplication on the parentheses term:

`\begin{gathered} y+4=(2)/(3)x+(2)/(3)\cdot6 \\ y+4=(2)/(3)x+4 \end{gathered}`

-Pass "+4" to the right side of the equation by applying the opposite operation "-4" to both sides of it:

`\begin{gathered} y+4-4=(2)/(3)x+4-4 \\ y=(2)/(3)x \end{gathered}`

The next step is to choose two values of x and replace them in the formula to determine the coordinates for both additional points, I will use x=3 and x=-3

1) For x=3

`\begin{gathered} y=(2)/(3)x \\ y=(2)/(3)\cdot3 \\ y=2 \end{gathered}`

The coordinates are: (3,2)

2) For x=-3

`\begin{gathered} y=(2)/(3)x \\ y=(2)/(3)(-3) \\ y=-2 \end{gathered}`

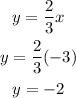
The coordinates are: (-3,-2)

Now you can graph the line, plot the coordinates of the three points (-6,-4), (-3,-2), and (3,2) in the coordinate system, then link them with a straight line: