Question

10 people meet, and each person shakes every other person's hand once. How many handshakes occurred?

Answer 1

45 handshakes would take place! i've attached how i figured this out (pardon the scribbles)

Answer 2

45 handshakes

Explanation:

If there are n persons and each person shakes every other person hand once then the total number of handshakes are
`(n(n-1))/(2)`

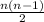
Now, it has been given that 10 people meet, and each person shakes every other person's hand once.

Therefore, n = 10.

Hence, from the above mentioned formula, we have

Total number of handshakes =

`(n(n-1))/(2)`

`=(10(10-1))/(2)`

`=(10*9)/(2)`

`=5*9`

`=45`

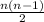
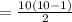
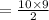
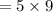
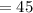
Hence, total 45 handshakes were occurred.