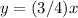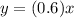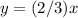Question

Relationship B has a greater rate than Relationship A. The graph represents Relationship A.

Which equations could represent Relationship B?

Choose all answers that are correct.
A. y = 3/4x

B. y = 0.6x

C. y = 2/3x

D. y = 1/4x

Help Meh

Answer 1

A,B,and C

I took the test but if you want a Step-by-step explanation comment below!

Answer 2

we know that

The rate of the Relationship is the slope of the function

so

Find the slope of the Relationship A

the slope is equal to

`m=((y2-y1))/((x2-x1))`

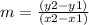
we have the points

`(4,2)\ and\ (8,4)`

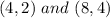
Substitute in the formula above to find the slope

`m=((4-2))/((8-4))`

`m=(2)/(4)=0.5`

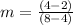
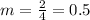
Compare the value of the rate of the Relationship A with each case

case a)

`y = (3/4)x`

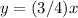
In this case the rate of the equation is equal to
`(3)/(4)`

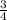
Compare with the rate of the Relationship A

`(3)/(4)>(2)/(4)`

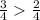
therefore

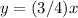`y = (3/4)x` -----> This equation could represent Relationship B

case b)

`y = (0.6)x`

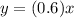
In this case the rate of the equation is equal to
`0.6`

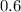
Compare with the rate of the Relationship A

`0.6 > (2)/(4)`

`0.6 > 0.5`

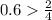
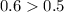
therefore

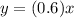`y = (0.6)x` -----> This equation could represent Relationship B

case c)

`y = (2/3)x`

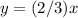
In this case the rate of the equation is equal to
`(2/3)`

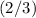
Compare with the rate of the Relationship A

`(2)/(3) >(2)/(4)`

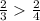
Multiply by
`12` both sides

`8 > 6`

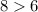
therefore

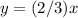`y = (2/3)x` -----> This equation could represent Relationship B

case d)

`y = (1/4)x`

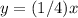
In this case the rate of the equation is equal to
`(1/4)`

Compare with the rate of the Relationship A

`(1)/(4) <(2)/(4)`

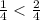
therefore

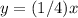`y = (1/4)x` -----> This equation could not represent Relationship B

therefore

the answer is

`y = (3/4)x`

`y = (0.6)x`

`y = (2/3)x`