Question

Hi! I need help with the attached question in calc. Thank you:)

Answer 1

3π square units.

Explanation:

We can use the disk method.

Since we are revolving around AB, we have a vertical axis of revolution.

So, our representative rectangle will be horizontal.

R₁ is bounded by y = 9x.

So, x = y/9.

Our radius since our axis is AB will be 1 - x or 1 - y/9.

And we are integrating from y = 0 to y = 9.

By the disk method (for a vertical axis of revolution):

`\displaystyle V=\pi \int_a^b [R(y)]^2\, dy`

So:

`\displaystyle V=\pi\int_0^9\Big(1-(y)/(9)\Big)^2\, dy`

Simplify:

`\displaystyle V=\pi\int_0^9(1-(2y)/(9)+(y^2)/(81))\, dy`

Integrate:

`\displaystyle V=\pi\Big[y-(1)/(9)y^2+(1)/(243)y^3\Big|_0^9\Big]`

Evaluate (I ignored the 0):

`\displaystyle V=\pi[9-(1)/(9)(9)^2+(1)/(243)(9^3)]=3\pi`

The volume of the solid is 3π square units.

Note:

You can do this without calculus. Notice that R₁ revolved around AB is simply a right cone with radius 1 and height 9. Then by the volume for a cone formula:

`\displaystyle V=(1)/(3)\pi(1)^2(9)=3\pi`

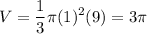
We acquire the exact same answer.