Question

One positive integer is 4 less than twice another. The sum of their squares is 544. Find the integers.

Answer 1

Taking x and y as the integers.

Let's say that x is 4 less than twice y. This is:

`x=2y-4`

The sum of the squares of x and y is 544, this is:

`x^2+y^2=544`

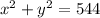
Use the first equation and replace this expression for the value of x in the second equation:

`\begin{gathered} (2y-4)^2+y^2=544 \\ 4y^2-16y+16+y^2=544 \\ 5y^2-16y+16-544=0 \\ 5y^2-16y-528=0 \end{gathered}`

Solve the quadratic equation

`\begin{gathered} y=\frac{16\pm\sqrt[]{16^2-4(5\cdot-528)}}{2\cdot5} \\ y=(16\pm104)/(10) \\ y1=(16+104)/(10)=(120)/(10)=12 \\ y2=(16-104)/(10)=-(44)/(5) \end{gathered}`

y can take 2 values, but, as we know, x and y must be positive integers, the value of y that meets this condition is 12. It means one of the integer is 12.

Use this value and the first equation to find the second integer:

`\begin{gathered} x=2y-4 \\ x=2\cdot12-4 \\ x=24-4 \\ x=20 \end{gathered}`

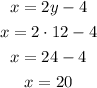
The integers are 20 and 12.