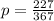Question

A hair salon completed a survey of 367 customers about satisfaction with service(Dissatisfied, Neutral, Satisfied, or Very Satisfied) and type of customer (Walk-in, sawa TV ad, or Referred). The results are summarized in the table below.

Answer 1

The results of the survey are shown in the table.

It's required to calculate the following probabilities:

a) A customer is neutral

The row labeled as 'Neutral' has a total of 79 customers out of 367 in total.

The required probability is:

`p=(79)/(367)`

b) A customer is a walk-in

The first column labeled as 'Walk-in' has a total of 106 customers out of 367. The required probability is

`p=(106)/(367)`

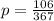
c) A customer is dissatisfied and a walk-in.

We have to cross the row Dissatisfied with the column Walk-in. The number of customers that have both categories is 22 out of 367.

The required probability is:

`p=(22)/(367)`

d) A customer is very satisfied given he saw a TV ad.

This is a conditional probability, where the total is 111 because is the given condition. The customers that have both characteristics are 33, thus the required probability is:

`p=(33)/(111)=(11)/(37)`

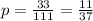
e) A customer is satisfied or referred.

Total satisfied = 139

Total referred = 150

With both features = 62

Total customers with one or both features: 139 + 150 - 62 = 227

We have to subtract the common feature because it was counted twice.

The required probability is:

`p=(227)/(367)`