Question

Two similar cylinders have a scale factor of (1.6/3). Find the ratio of their volumes.

Answer 1

Volume of cylinders = pi x r^2 x height

Simplifying the ratio:
1.6/3 = 16 / 30 (multiply and divide by 10 to remove the decimal)

Volume Ratio = 512:27

Answer 2

Answer: The ratio of the volumes of the two cylinders is 512 : 3375.

Step-by-step explanation: Given that two similar cylinders have a scale factor of
`(1.6)/(3).`

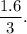
We are to find the ratio of the volumes of the two cylinders.

We know that

the volume of a cylinder with radius r units and height h units is given by

`V=\pi r^2h.`

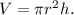
Let r and R be the radii and h and H be the heights of the two cylinders.

Then, we must have

`(R)/(r)=(1.6)/(3)=(16)/(30)=(8)/(15)\\\\\Rightarrow R=(8)/(15)r.`

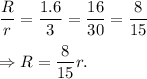
And, similarly,

`H=(8)/(15)h.`

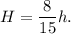
Therefore, the volume of the first cylinder will be

`V_1=\pi r^2h`

and volume of the second cylinder will be

`V_2=\pi R^2H=\pi*\left((8)/(15)r\right)^2*\left((8)/(15)h\right)=(512)/(3375)\pi r^2h.`

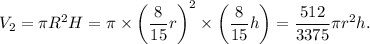
Thus, the required ratio of the volumes of the two cylinders is given by

`(V_2)/(V_1)=((512)/(3375)\pi r^2h)/(\pi r^2h)=(512)/(3375)=512:3375.`

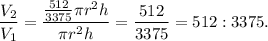
The ratio of the volumes of the two cylinders is 512 : 3375.