Question

This is to Represent the point of G.

Answer 1

Answer: (11,10)

======================================================

Step-by-step explanation:

The notation
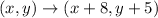`(x,y) \to (x+8, y+5)` means that we add 8 to the x coordinate and add 5 to the y coordinate. This represents a shift of 8 units to the right and a shift of 5 units up.

Point G is located at (3,5). This means x = 3 and y = 5 pair up together.

Using the translation rule, we get the following

`(x,y) \to (x+8, y+5)\\\\(3,5) \to (3+8, 5+5)\\\\(3,5) \to (11, 10)\\\\`

Point G at the location (3,5) moves to point G' which is at the location (11,10)