Question

I'm not sure on how to get P(Z < 1.555) from 6%Suppose the time to complete a 200-meter backstroke swim for female competitive swimmers is normally distributed with a mean μ = 141 seconds and a standard deviation σ = 7 seconds. Suppose the fastest 6% of female swimmers in the nation are offered college scholarships. In order to be given a scholarship, a swimmer must complete the 200-meter backstroke in no more than how many seconds? Give your answer in whole numbers.

Answer 1

`130\text{ seconds}`

Step-by-step explanation:

Here, we want to get the time a swimmer must complete the backstroke, in order to be considered for a scholarship

What we need to find here is the time in which the probability would be less than 6% (or 6/100 = 0.06)

Now, let us get the z-score to the right of 0.06 but closest to it

By using the normal distribution table, we have this value as:

`1.55\text{ SD below the mean}`

Recall, mathematically:

Substituting the values, we have it that:

`\begin{gathered} -1.55\text{ = }(x-141)/(7) \\ x\text{ = 141-7(1.55)} \\ x\text{ = }130.15\text{ } \end{gathered}`

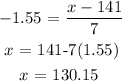
The value of x can be approximated as 130 seconds