Question

In the two similar trapezoids below, HE = 20, what is DA?

A. 10

B. 20

C. 30

D. 40

Answer 1

Esta es la respuesta. C 30

Answer 2

DA = 30

Explanation:

Given : the two trapezoid AFGH and ABCD are similar.

We have to find the value of DA , given HE =20

Two figures are said to be similar if their corresponding sides are in same ratios.

The two given trapezoid AFGH and ABCD are similar.

Then their corresponding sides are in same ratios that is

`(HE)/(DA)=(HG)/(DC)=(GF)/(CB)=(EF)/(AB)`

Substitute the values for given sides , we have,

`(20)/(DA)=(56)/(42x)=(10x)/(30)=(EF)/(70)` .....(1)

Now , first we find the value of x,

Comparing second and third ratio, we have,

`(56)/(42x)=(10x)/(30)`

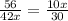
Cross multiply, we get

`56 \cdot 30=10x\cdot 42x`

Simplify for x, we have,

`56=14x^2`

`x^2=4`

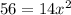
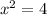
Thus, x = 2 as x can not be negative.

Then (1) becomes,

`(20)/(DA)=(56)/(84)=(20)/(30)=(EF)/(70)`

Comparing first and third ratio, we get,

`(20)/(DA)=(20)/(30)`

Now solve for DA,

we get DA = 30

Thus, DA = 30