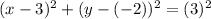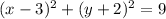Answer: B.
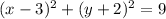
Explanation:
From the given graph, it can be seen that the center of circle lies at (3,-2).
Radius = distance from the center to the boundary of circle.
=|3-0|=3 units
Standard equation of circle :
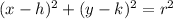 , where (h,k) is center and r is the radius of the circle.
, where (h,k) is center and r is the radius of the circle.
Then , standard equation of the circle in the graph (having center (3,-2) and radius = 3 units)